A Teacher Affects Eternity—And So Do His Kids

By Laurie Maloff Kramer

Create a sense of wonder about mathematics. Challenge high school students with logic and math problems that stray far from the standard curriculum and make them think outside their comfort zone.

Ira Ewen, just a few years out of Harvard College, followed that teaching philosophy 60 years ago at a New York City public high school—not a selective school like Stuyvesant or Bronx Science, but a neighborhood school in Queens with the unlikely name of Martin Van Buren. He taught students who went on to achieve at the highest levels in math, computer science, and physics.

Jeff Ullman, a professor emeritus of engineering at Stanford, won the A.M. Turing Award in 2020, considered the equivalent of the Nobel Prize in computer science. The Turing Award cited Ullman and his coauthor for their “highly influential books, which educated generations of computer scientists.”

*Compilers*, known as the *Dragon Book*, co-authored by Jeff Ullman—one of the “highly influential books which educated generations of computer scientists” according to the 2020 Turing Award.

Frank Wilczek, a professor of theoretical physics at MIT, won a MacArthur Fellowship in 1982, the Nobel Prize for Physics in 2004, and the Templeton Prize in 2022.
Joel Spencer and Dan Fendel both became Putnam Fellows, outscoring the best undergraduate mathematicians in the country. Spencer became a professor of computer science and math at Stony Brook and NYU and an internationally recognized authority on Ramsey Theory. He co-authored The Probabilistic Method, winner of the 2021 Steele Prize for Mathematical Exposition. Fendel became a math professor at San Francisco State and co-authored The Interactive Mathematics Program, an award-winning high school math curriculum.

*The Probabilistic Method* co-authored by Joel Spencer—winner of the 2021 Steele Prize for Mathematical Exposition. The cover photo shows Paul Erdos, creator of the method.

These were among the many students inspired by Ewen. As math and science professors, they went on to inspire their own students, in the generational chain envisioned by Henry Adams when he famously said, “A teacher affects eternity; he can never tell where his influence stops.”

Saturdays with Ira—on Zoom

Ira Ewen at his 70th Harvard reunion, June 2022. Photo courtesy of Rani Ewen Dalgin.

Now 91, Ewen has a razor-sharp mind trapped in a disintegrating body. Following a broken hip last December, he moved from New York to a nursing home in Belmont, Mass. He spends his days in a recliner, doing cryptic crosswords, inventing logic puzzles, and playing bridge on the internet with top national and international players. He calls the bridge games his “only contact with the outside world.” He roots for the NY Mets, watching games with fanatic fervor. He rarely leaves the nursing home, but he did attend his 70th Harvard reunion in June.

Ewen reconnected with 21 of his former students—sixteen men and five women—during a quartet of Zoom calls this year.

Ira Ewen on the second Zoom call, July 2022. Photo by Laurie Kramer.

In the first call, he articulated his philosophy of education. “The sense of wonder is our most important gift—to have always, no matter how old you become and no matter how badly life has treated you. Remember that life is an adventure—and it will contain for each of us very great ups and very great downs.” The most important lesson any child can learn is not to be discouraged by failure but to go beyond that, he asserted. “When a child is taught by the parent that only success is to be rewarded it is a disastrous lesson. If the child is always looking only to do the things in which they succeed they will never attempt anything truly useful.”

In the other calls, the participants (the “kids”), mostly mathematicians or scientists now in their 70s, and teacher talked about weighty topics: the relationship between the Big Bang and the infinite universe, the nature of self-awareness, whether or not computers will ever think for themselves, Peano Postulates, the Butterfly Problem, Euclid's parallel postulate, infinitesimals, the Collatz conjecture, the existence of other intelligent life in the universe, and which game is hardest—bridge, chess or Go. Ewen happily explained that “unlike chess and Go, bridge has not been mastered by computers and so remains the hardest game.”

50+ Years Ago: A Portrait of the Teacher as a Young Man

Ira Ewen at Martin Van Buren High School, 1965. Photo from *Futura.*

Sitting in Mr. Ewen’s class, I often felt I was in the presence of a genius. I wasn’t the only one. At the time, we never asked him why a brilliant mathematician became a high school teacher.

Ewen did begin PhD studies in math at Harvard but left before finishing his dissertation. His daughter recently said that he made a “personal decision” not to pursue a doctorate. He “wanted to teach and felt it was important to do it at the high school level.”

Whatever brought Ewen to high school teaching, his students were the grateful beneficiaries.

Physicist Larry Jackel describes his impressions of Mr. Ewen at Van Buren: “He was imposing. He walked with authority: a young version of Dumbledore without the folderol. Mr. Ewen taught math classes with the rigor you do not usually see until advanced courses in college. . . . [he] tasked us with problems like this: You have a quart jar half filled with a liquid that is 30% essence of cat. How much water do you have to add to have a liquid that is 20% essence of cat? This is the kind of nuttiness I later discovered was common in many math and science types.”

College professor Hal Gabow is a lifelong fan of Mr. Ewen’s “teaching gems.” Explaining commutativity, for example, a traditional teacher might simply say that addition is commutative because a+b equals b+a, and subtraction isn't commutative because a-b does not equal b-a. Not Mr. Ewen. “Mr. Ewen stood in front of the class and jumped up, saying ‘If I jump forward and then walk to the window, it's not the same as if I walk to the window and then jump forward,’” Gabow recalls.

In 1964, Ewen created an enhanced bridge and chess club for students interested in mathematical games. “I had no interest in either game, but that was where Mr. Ewen held court, so I hung out there too. I can’t recall precisely what he said on those Fridays, but a bunch of us were enthralled by his brilliance, humor, and decency,” Jackel reminisced.

Warm as his relations were with students, Ewen now says openly that he did not get along well with the rest of the Van Buren math department. Even those teachers still remembered by former students as excellent wanted everyone to stick to the standard curriculum, he said. But Ewen wanted to teach not just math, but the foundations and theory of math and how to “look on unfamiliar problems in productive ways.” He instructed every student: “Don’t ask what the answer is—tell me what the question means.” Maybe his teaching philosophy was just too unusual for the time, and they weren’t having any of it.

A Problem-Based Approach to the Teaching of Mathematics

In the late 1960s, Ewen became the founding math department chair at John Dewey High School, a new school opening in Brooklyn, NY. Faced with the challenge of staffing a whole department, he asked candidates this question during interviews: “What math problem are you working on now?” If they gave him a puzzled look, the interview was over. If they explained with enthusiasm the problem they were working on, he hired them.

In his own teaching, Ewen was known for challenging students with unfamiliar problems. In early 2022 on The Ramsey Theory Podcast: No Strangers at This Party with Joel Spencer, Spencer reminisced: “In high school I had this incredible, very inspirational teacher [Ira Ewen] who showed me what mathematics was and was really critical to my development.” Ewen gave him this challenge: If you have six points and you draw the 15 lines connecting pairs of points and color the lines red or blue, PROVE that there must be a monochromatic triangle (an all-red triangle or an all-blue triangle). “I was an energetic high school student and so I worked and worked. Finally I came to him with a 20-page proof that showed that no matter how you color the lines, in all the cases you wind up getting either a red triangle or a blue triangle. And then he showed me the proof that….is the beginning of Ramsey Theory, and I was smitten by the beauty of this argument.” Spencer adds: “I’ve read reports of other mathematicians who have recalled working for ages and ages on a problem and then were shown a beautiful proof and somehow that got them hooked. And that was the case for me.”

Ewen still delights in discussing interesting problems. He posed some of his favorites during recent Zoom calls. Professor Jeff Ullman introduced two of those problems to top international math and computer science students at the 9th Heidelberg Laureate Forum, September 18-23, 2022. “Not only did many of the students dig into the problems immediately,” Ullman reported from Heidelberg, “but these problems were passed around to the extent that students I never met are coming up and asking about them.” Below are the problems with warm-up puzzles added to help you get started. Bring these problems into your classroom and see what a lasting impact it may have on your students.

Epilogue

The third Zoom call, August 2022. Left to right, top to bottom: Ira Ewen, Laurie Kramer, Harold Gabow, Marvin Zelkowitz, Bob Shishko, Dennis Drapkin, Bill Anscher, Frank Wilczek. Photo by Laurie Kramer.

Talking to each other via Zoom, Ewen thanked the students for the best gift he’d received in decades: the gift of their appreciation, “a star in a darkened universe.” Just like the old days, he challenged them with numerous tough math and logic problems. The students thanked Ewen for brilliant and creative teaching—and for the mentoring that changed the trajectories of their lives. Then they signed off and started working on the problems.

Laurie Maloff Kramer graduated from Martin Van Buren High School in 1965 and from Smith College in 1969. She is author/compiler of Back in the Day—Martin Van Buren High School: The Early Years 1955-1971, published by the school’s Alumni Alliance in 2022. Thanks to Professors Jeff Ullman and Hal Gabow for their help with this article.

Some Logic Problems to Wrap Your Head Around

Problems labeled warm-up are courtesy of Professors Jeff Ullman and Hal Gabow. Problems labeled harder are courtesy of Ira Ewen.

CONSONANTS: What word in English contains the consonants “WSST” in that order in the middle of the word with no vowels in between the consonants? What about the consonants “CHSH”? What unhyphenated word in English contains “WW”?
CHECKERBOARDS: Warm-up. Using a standard checkerboard of 8x8 squares, remove two diagonally opposite squares. Can you cover the board completely with standard dominoes? Harder. Take a standard checkerboard of 8x8 squares. You can cover it completely with 21 rectangular pieces sized 3x1 (covering three squares on the board) and one piece that’s 1x1 (covering one square on the board). There are four squares where the 1x1 piece can go. Which are they and why don’t any others work?
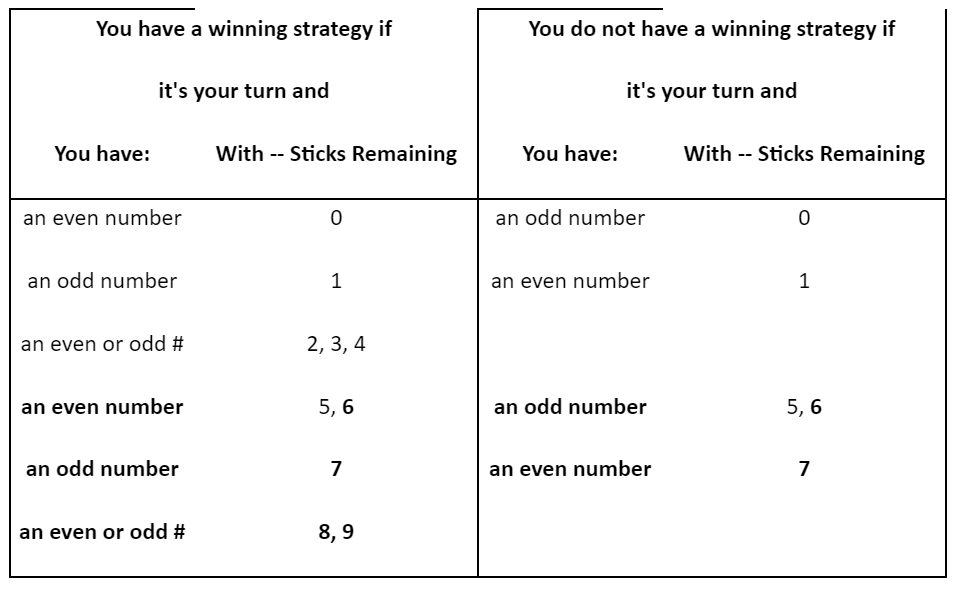
MATCHSTICKS: This is a game for two players. Start with 27 matchsticks. Each player can remove 1, 2, 3 or 4 matchsticks per turn. Warm-up. The goal is to take the last matchstick. Who can always win—the player going first or the player going second? Harder. The goal is to end with an even number of matchsticks. Is it better to go first or second in the game?
TRUTH/LIES: This classic puzzle has many variations. Warm-up. An explorer is in the jungle and comes to two paths. One path leads to a cannibal village and one leads to a safe place. There's a tribesman standing there, and the explorer knows that this tribesman knows which path is which. But the explorer doesn't know whether the tribesman comes from a tribe which always tells the truth or which always lies. What ONE yes/no question can the explorer ask this tribesman which will reveal the safe path, no matter whether the tribesman is a truth teller or a liar? Harder. The explorer is again in the jungle and comes to the same two paths. But this time there are three tribesmen standing there each wearing a distinctive costume from a different tribe. And the explorer knows that one of the tribes always tells the truth, one of the tribes always lies, and one of the tribes answers randomly to any yes/no question. Can the explorer, in TWO yes/no questions, find out which path is safe?
HEADS/TAILS: Warm-up. When tossing a fair coin, what is the probability of getting one head before four consecutive tails? Harder. What is the probability of getting two consecutive heads before getting four consecutive tails?

Solution Sketches to the Harder Versions

CONSONANTS:

WSST: newsstand CHSH: dachshund WW: powwow

CHECKERBOARDS:

Draw a colorless 8x8 checkerboard, and color the diagonals alternately red, white, and blue. Every 3x1 rectangle (two standard dominoes) will cover squares of all 3 colors. One color, say blue, has 22 squares, the other two have 21. Only 1 set of 4 symmetric squares is all blue.

Partial solution to puzzle number 2, the harder version (see Sidebar). Photo courtesy of Hal Gabow.

MATCHSTICKS:

At the end of the game, when zero sticks remain, a player wins if she holds an even number of sticks and loses if she holds an odd number of sticks. Also, when one stick remains and it's her turn, a player wins if she holds an odd number of sticks and loses if she holds an even number of sticks. This gives the first four entries in a table giving winning and losing positions.

The bold entries for 6, 7, 8, and 9 duplicate the first four table entries, for 0, 1, 2, and 3. Since you can take at most 4 sticks in one move, each subsequent entry, for 10, 11, and so on, must agree with the entry for the number six below it. So we can extend the table by adding 6 to every entry. Thus, the entry for 25 will be the same as the entry for 19, 13, 7, and 1; i.e., you win if you have an odd number (if it is your turn) and lose if you have an even number. Since your opponent has an even number of sticks (zero) to begin, the first player wins by choosing 2 sticks, leaving their opponent with 25 remaining on the board.

TRUTH/LIES:

See https://suresolv.com/brain-teaser/liar-truth-teller-random-answerer-riddle-step-step-solution.

HEADS/TAILS:

Get to the first H flipped via H, TH, TTH, or TTTH, probability 1/2+1/4+1/8+1/16 = 15/16.

Let p = probability of success starting from a flip of H.

Then p = prob(H) + prob(TH or TTH or TTTH) p

= 1/2 + (1/4 + 1/8 + 1/16)p.

So (9/16)p = 1/2. Thus, p = 8/9.

Overall probability = (15/16) (8/9) = 5/6.