The Shifting Sands of Algebra

By Keith Devlin @KeithDevlin@fediscience.org, @profkeithdevlin.bsky.social

My August post about the growth (and changing nature) of algebra generated some feedback. For the most part, those who objected to one or more statements seemed to be motivated by an assumption of what the word “algebra” means (or should be taken to mean).

That certainly seems to be at the root of comments that I went too far with my statements “[A]lgebra is the language of science and engineering” and my allusion to the new Counted Out movie (the subject of my July post), which makes the case that citizens having some mastery of algebra is as important in today’s world as mastery of our native human language.

Coming from university mathematicians, I suspect those respondents were using “algebra” in the sense assumed in university “algebra courses” for mathematics majors and graduate students.

Other university mathematicians took issue with my inclusion of the introductory remark K-12 teachers sometimes use to introduce algebra, as “arithmetic done backwards”.

Of course, university mathematics professors and K-12 mathematics teachers have a professional interest (indeed, arguably a duty) to use the word “algebra” in a consistent way, to mean what it is they are teaching. (University engineering professors likely have a somewhat different meaning in mind than their colleagues in the mathematics department, and they would likely resonate with my statement, but they probably don’t read my column.)

But having just completed a new “general reader” book on the history of algebra (co-written with a historian; hopefully it will appear next year), I was adopting as broad a meaning as possible, and definitely one that encompasses all the ways the word is used today. (Hence my quotation of dictionary definitions in my essay.)

The point is, the algebra we are all familiar with today is just over a century old. Moreover, it is built on a concept of number and a number system (actually, number systems, in the plural) that are themselves products of the early Twentieth Century. And it’s likely not the meaning we associated with the word after we took our first algebra class in school.

The modern framework we (or at least, many of us, including writers of dictionaries) refer to as “algebra” is so powerful, both expressively and computationally, that we can apply it to the entire canon of historical works and at once understand them. They look modern.

And it is through that lens that we typically approach the history of mathematics.

It’s a powerful approach, but a dangerous one. For what it yields is a modern-day description of processes that in a very general way parallel what our mathematical ancestors did, but in point of fact differs in almost every way from what they were actually doing.

In particular, and of significance, that parallel could not run the other way. If we were to time-travel back and meet al-Khwārizmī, or Fibonacci, or Descartes and try to describe modern algebra, it would take them some time, and a lot of help, to understand what we do today. (Yes, they were very smart, so presumably capable of coming quickly to mastery, but I’m not entirely sure of this.)

Certainly, if you read Newton’s notebooks (written without a time-traveler from 2024 to help) and see his attempts to define a concept of number that would support his new calculus, you realize why it took until the end of the Nineteenth Century to develop the modern number system. The mathematics we accept as natural today, and teach to young children, is the product of two thousand years of human development. It’s a colossal cultural achievement.

The very length of time it took to get to where we are today, should make us realize what a difficult task it was to progress mathematically. As I tried to argue years ago in my 2001 book The Math Gene, mathematical thinking did not come easily to the human brain. Recognizable advances are generally phase-shifts resulting from many generations of tiny changes in how we think.

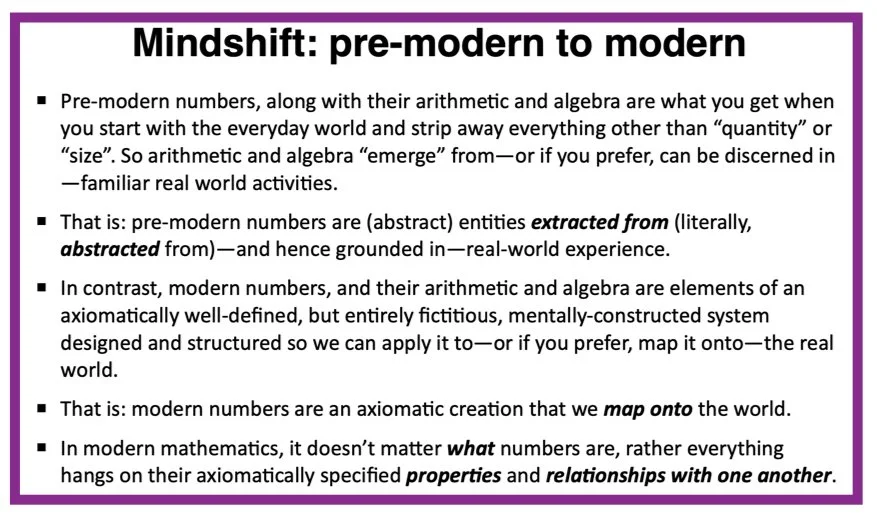
I tried to capture this cognitive-evolutionary process in a single slide for a course on the History of Mathematics for future school teachers in Denmark that I am (at the time of writing) co-teaching over Zoom. (There’s a professor physically present in the room at Southern Denmark University in Odense.) I’ll leave you with that image.

If you detect echoes of Lakoff and Núñez’s book Where Mathematics Comes From in that slide, you are right. I don’t agree with every point they make, particularly later in the book, but overall I love it. (It appeared around the same time as my The Math Gene, from the same publisher, and starts off pretty well where my book ends, and I knew/know both authors, but there was no coordination between us or even knowledge of what the other was up to.)

But maybe that coincidence should not be so surprising. I’ll take this opportunity to pass on an excellent little essay my SDU-co-instructor directed the students to, regarding “academic coincidences”. This one being the infamous Newton-Leibniz dispute about the invention of calculus.

By the way, the Danish education system requires all future mathematics teachers to take a course on the history of mathematics as part of their obligatory education masters degree. I like that.